Freatic groundwater levels benchmark (Water Module): Difference between revisions
No edit summary |
No edit summary |
||
| (17 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
This | This [[Water Module benchmarks|benchmark]] demonstrates a situation where a parcel of land is situated between two waterways, each with a stable but different water level. In combination with continuous rainfall, a characteristic curve will form over time, as shown in the image below. | ||
[[File:freatic_rain_fall.gif|A parcel of land situated between two waterways during continuous rainfall]] | [[File:freatic_rain_fall.gif|A parcel of land situated between two waterways during continuous rainfall]] | ||
| Line 7: | Line 7: | ||
Note that the time at which this balance occurs is dependent on the starting situation. | Note that the time at which this balance occurs is dependent on the starting situation. | ||
The following formula <ref name="Bear79"/> describes the curve when the ground water | The following formula, taken from <ref name="Bear79"/>, describes the curve of ground water levels when the ground water flow to the left and right have become stable: | ||
<math> h^2 (x) = h_0^2 - (\frac{h_0^2-h_L^2}{L}) x + \frac{N}{k} (L- x) x</math> | <math> h^2 (x) = h_0^2 - (\frac{h_0^2-h_L^2}{L}) x + \frac{N}{k} (L- x) x</math> | ||
| Line 19: | Line 20: | ||
===Setup=== | ===Setup=== | ||
The following setup has been taken from [http://grondwaterformules.nl/index.php/formules/peilverschil/rechte-strook-freatisch grondwaterformules.nl]. | The following setup has been taken from the use case ''Peilverschil over een strook grond (freatisch)'' at [http://grondwaterformules.nl/index.php/formules/peilverschil/rechte-strook-freatisch grondwaterformules.nl]. | ||
In the situation described there, the chosen Length L is set to 500 m. This is achieved with a grid size of 28 by 5, with a cell size of 20 m. There are two stable ground water levels, one on left of 11 meters (datum) and one on the right at 10 meters (datum). The rainfall is 0.8 | In the situation described there, the chosen Length L is set to 500 m. This is achieved with a grid size of 28 by 5, with a cell size of 20 m. There are two stable ground water levels, one on left of 11 meters (datum) and one on the right at 10 meters (datum). The rainfall is 0.8 mm per day, the horizontal infiltration speed is 3 m per day. | ||
| Line 32: | Line 33: | ||
Additionally, two [[Inlet (Water Overlay)|underground inlets]] are placed, one on x=1 and one on x=26, as an area over y = 1 to 3. | Additionally, two [[Inlet (Water Overlay)|underground inlets]] are placed, one on x=1 and one on x=26, as an area over y = 1 to 3. | ||
The simulation time is set to | The simulation time is set to n days, with a rainfall of 0.8 mm per day. To configure this, the [[Weather rain m (Water Overlay)|rain]] set is set to <math>[3600 \cdot 24 \cdot n, \frac{0.8}{1000 \cdot 3600 \cdot 24}]</math>. | ||
===Results=== | ===Results=== | ||
====365 days==== | |||
The first result is generated for n = 365:<br> | |||
[[File:benchmark_freatic_365.png]] | |||
====730 days==== | |||
The second result is generated using n = 730:<br> | |||
[[File:benchmark_freatic_730.png]] | |||
===Notes=== | |||
* The amount of days it takes to reach the stable solution is highly dependent on the starting situation. | |||
===References=== | ===References=== | ||
Latest revision as of 14:48, 5 March 2024
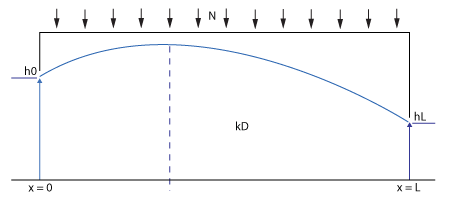
This benchmark demonstrates a situation where a parcel of land is situated between two waterways, each with a stable but different water level. In combination with continuous rainfall, a characteristic curve will form over time, as shown in the image below.
Formulas
Due to continuous rainfall and two stable water levels left and right, a specific ground water table curve will form. Part of the rainwater will flow left and part of the it will flow right. Note that the time at which this balance occurs is dependent on the starting situation.
The following formula, taken from [1], describes the curve of ground water levels when the ground water flow to the left and right have become stable:
where:
- : distance of the left waterway edge (m)
- : water level at x = 0 (m)
- : water level at x = L (m)
- : distance between both waterways (m)
- : horizontal infiltration speed (m / day)
- : additional ground water (m/day).
Setup
The following setup has been taken from the use case Peilverschil over een strook grond (freatisch) at grondwaterformules.nl.
In the situation described there, the chosen Length L is set to 500 m. This is achieved with a grid size of 28 by 5, with a cell size of 20 m. There are two stable ground water levels, one on left of 11 meters (datum) and one on the right at 10 meters (datum). The rainfall is 0.8 mm per day, the horizontal infiltration speed is 3 m per day.
The terrain height is set to 12 for all cells and the ground bottom distance is also set to 12.
The terrain type's infiltration speed can be configured to 3 m / day.
For x = 1 the initial ground water level is set to 11 and for x = 26 the ground water level is set to 10. The initial ground water levels between this points is linearly interpolated.
Additionally, two underground inlets are placed, one on x=1 and one on x=26, as an area over y = 1 to 3.
The simulation time is set to n days, with a rainfall of 0.8 mm per day. To configure this, the rain set is set to .
![{\displaystyle [3600\cdot 24\cdot n,{\frac {0.8}{1000\cdot 3600\cdot 24}}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6cffc8c3e1d93611fec069d30bad8307c9e55afd)
Results
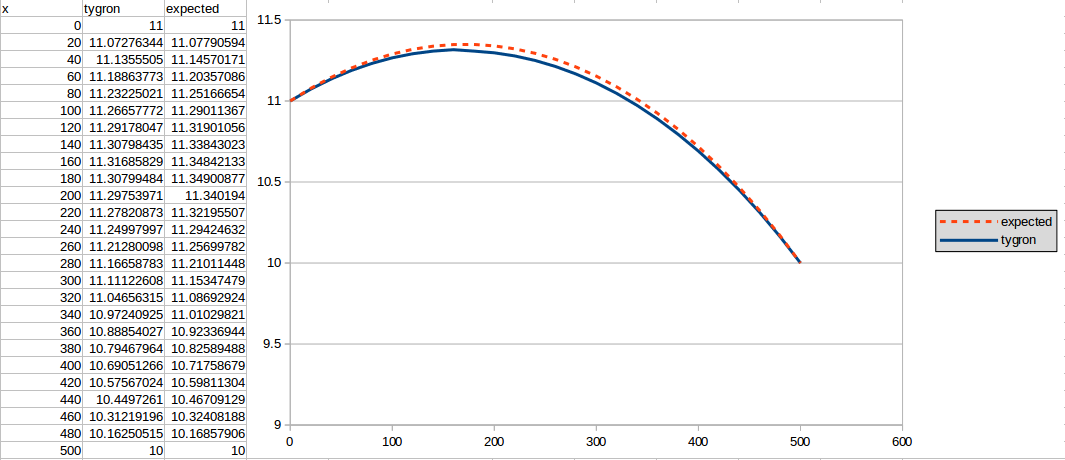
365 days
The first result is generated for n = 365:
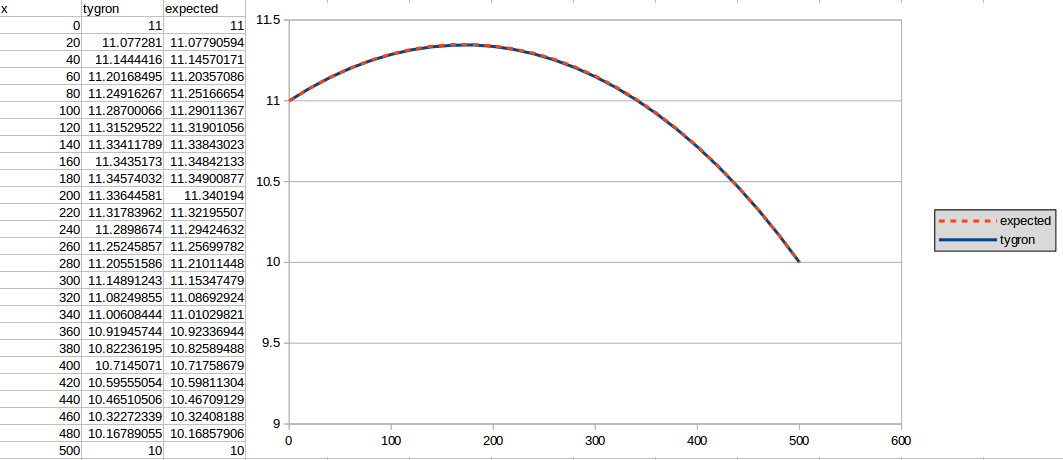
730 days
The second result is generated using n = 730:
Notes
- The amount of days it takes to reach the stable solution is highly dependent on the starting situation.
References
- ↑ Bear, J., 1979. Hydraulics of Groundwater. McGraw-Hill. (formula on p.180, equation 5-212)
